Plastic Analysis
Plastic Analysis
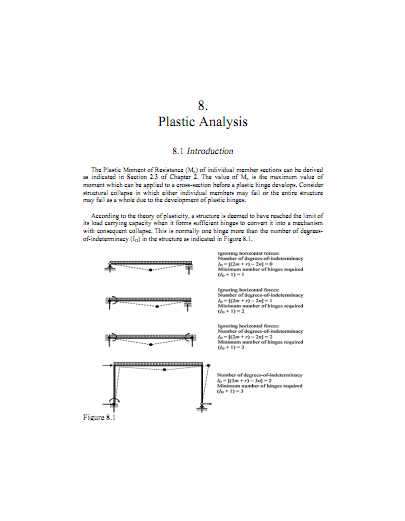
8.1 Introduction
The Plastic Moment of Resistance
of individual member sections can be derived as indicated in Section 2.3 of Chapter 2. The value
is the maximum value of moment which can be applied to a cross-section before a plastic hinge develops. Consider structural collapse in which either individual members may fail or the entire structure may fail as a whole due to the development of plastic hinges.
According to the theory of plasticity, a structure is deemed to have reached the limit of its load carrying capacity when it forms sufficient hinges to convert it into a mechanism with consequent collapse. This is normally one hinge more than the number of degreesof-indeterminacy
in the structure as indicated in Figure 8.1.

2.5 Plastic analysis
In plastic analysis and design of a structure, the ultimate load of the structure as a whole is regarded as the design criterion. The term plastic has occurred due to the fact that the ultimate load is found from the strength of steel in the plastic range. This method is rapid and provides a rational approach for the analysis of the structure. It also provides striking economy as regards the weight of steel since the sections required by this method are smaller in size than those required by the method of elastic analysis. Plastic analysis and design has its main application in the analysis and design of statically indeterminate framed structures.
2.5.1 Basics of plastic analysis
Plastic analysis is based on the idealization of the stress-strain curve as elastic-perfectly-plastic. It is further assumed that the width-thickness ratio of plate elements is small so that local buckling does not occur- in other words the sections will classify as plastic. With these assumptions, it can be said that the section will reach its plastic moment capacity and then undergo considerable rotation at this moment. With these assumptions, we will now look at the behaviour of a beam up to collapse.
Consider a simply supported beam subjected to a point load W at mid-
span. as shown in Fig. 2.14(a). The elastic bending moment at the ends is w
and at mid-span is w
/24, where is the span. The stress distribution across any
cross section is linear [Fig. 2.15(a)]. As W is increased gradually, the bending moment at every section increases and the stresses also increase. At a section
close to the support where the bending moment is maximum, the stresses in the extreme fibers reach the yield stress. The moment corresponding to this state is called the first yield moment M
, of the cross section. But this does not imply failure as the beam can continue to take additional load. As the load continues to increase, more and more fibers reach the yield stress and the stress distribution is as shown in Fig 2.15(b). Eventually the whole of the cross section reaches the yield stress and the corresponding stress distribution is as shown in Fig. 2.15(c). The moment corresponding to this state is known as the plastic moment of the cross section and is denoted by
. In order to find out the fully plastic moment of a yielded section of a beam, we employ the force equilibrium equation, namely the total force in compression and the total force in tension over that section are equal.
Download
*